| Pri 2,320t RLa-a |
Sec
51tpl, 4 // 51t, 3 // 68t |
Sec
54tpl, 4 // 54t, 3 // 72t |
Sec
57tpl, 4 // 57t, 3 // 76t |
Sec
60tpl, 4 // 60t, 3 // 80t |
||||
| 8k0 |
3r9
|
6r9 | 4r3
|
7r7 | 4r8**** |
8r6 | 5r4**** |
9r5 |
| 7k0 |
3r4 |
6r0 | 3r8 |
6r7 | 4r2 |
3r7 | 4r7**** |
8r3 |
| 6k0 |
2r9 |
5r2**** | 3r2 |
5r8 | 3r6 |
6r5 | 4r0 |
7r1 |
| 5k0 |
2r4 |
4r3 | 2r7 |
4r8**** | 3r0 |
5r4**** | 3r4& |
5r9 |
| 4k0 |
1r9 |
3r4 | 2r2 |
3r9 | 2r4 |
4r3 | 2r7 |
4r7**** |
| RLa-a 2,320t |
Ns 4 // 51t |
Ns 3 // 68t |
Ns 2 // 102t |
Po Max |
Va-a Vrms |
| 4k0 |
1r9 |
3r4 |
7r7 |
40W |
410 |
| 6k0 |
2r9 |
5r1 |
11r6 |
34W |
466 |
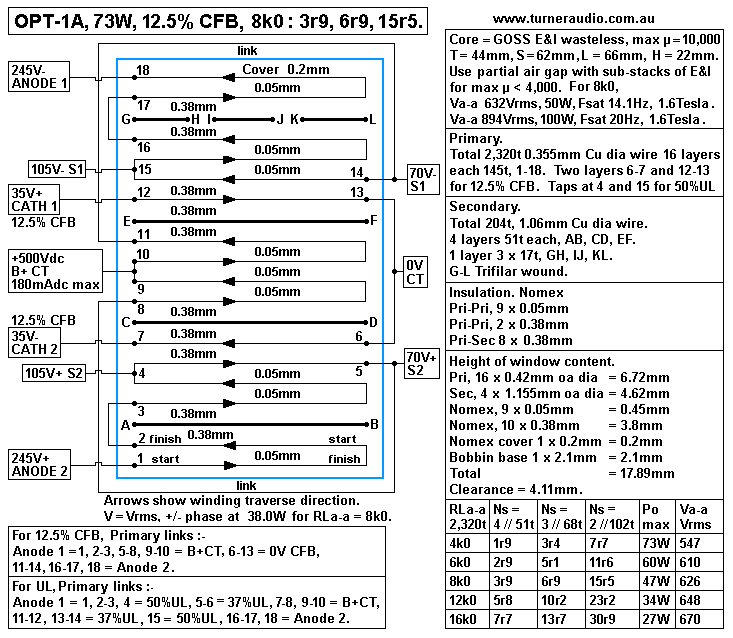
| 8k0 |
3r9 |
6r9 |
15r5 |
29W |
494 |
| 16k0 |
7r7 |
13r7 |
30r9 |
21W |
580 |
| La-a 2,320t |
Ns 4 // 51t |
Ns 3 // 68t |
Ns 2 // 102t |
Po Max |
Va-a Vrms |
| 4k0 |
1r9 |
3r4 |
7r7 |
160W |
820 |
| 5k0 |
2r4 |
4r3 |
9r6 |
135W |
848 |
| 6k0 |
2r9 |
5r1 |
11r6 |
121W |
876 |
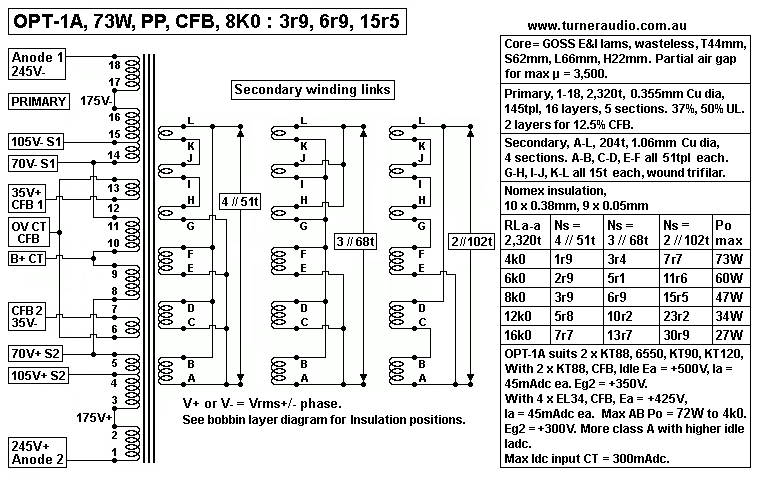
| 8k0 |
3r9+++ |
6r9+++ |
15r5+++ |
95W |
898 |
| 12k0 |
5r8 |
10r3 |
23r2 |
72W |
931 |
| 16k0 |
7r7 |
13r7 |
30r9 |
55W |
939 |
| Mode, all with
RLa-a 8k0, Low Va-a < 20Vrms |
Ra-a |
Ra-a // Ra-a |
F1 |
F2 |
| Pure Tetrode,
fixed Eg2 |
64k |
7k1 |
14.1Hz |
43kHz |
| 40% UL |
5k6 |
3k3 |
6.6Hz |
92.5kHz |
| Triode, g2 to
anode |
2k2 |
1k7 |
3.4Hz |
179kHz |
| 20% CFB, fixed
Eg2 |
1k5 |
1k3 |
2.6Hz |
466kHz. |
